How Do You Change A Negative Exponent To A Positive Exponent

Negative Exponents & the Decimal System

Today we're learning all near negative exponents. What are they? And how practice you handle them when they show up in algebraic expressions?

The Decimal Arrangement

In a previous lesson, I introduced the decimal system and exponential notation. Call up the decimal system is base 10, meaning we have 10 symbols and we assign value to the symbols based upon the symbol and its position.

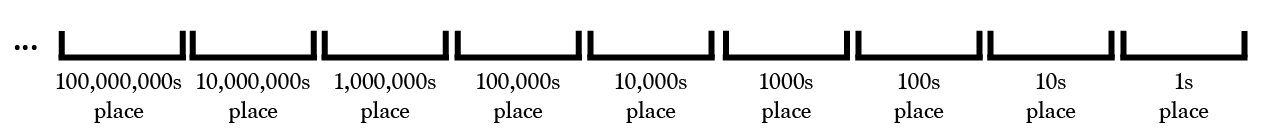
To tell you the truth, this is simply half of the story. Don't worry, math is inherently balanced, so the other half of the decimal organization is a elementary extension of what we accept above. Let's derive information technology.

Across the Decimal Point

Begin by mark the decimal point to the right of the i's place. The decimal point separates the whole number values on the left from the fractional values on the right.

Moving left-to-correct along the decimal identify-value system is equivalent to dividing by x each time. Keep this dividing procedure to the right of the decimal bespeak to obtain the residual of the decimal system.

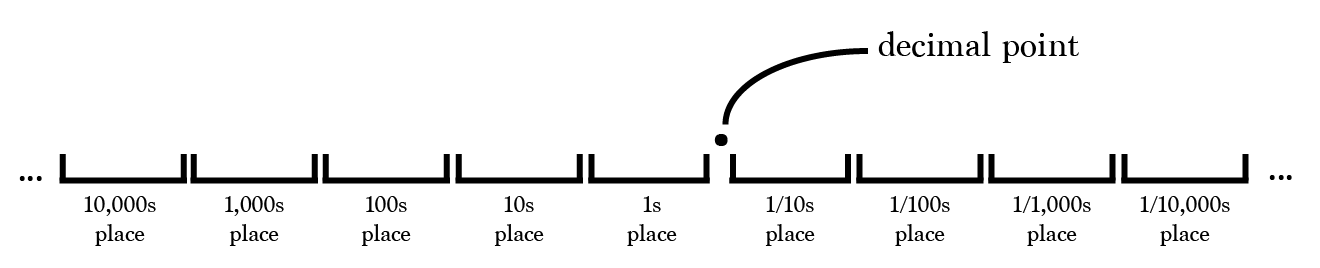
diagram 1: decimal system with numeric labels

Nosotros call these place-values: tenths, hundredths, thousandths, x-thousandths, etc.

Place-values on the left can be represented in powers of 10:

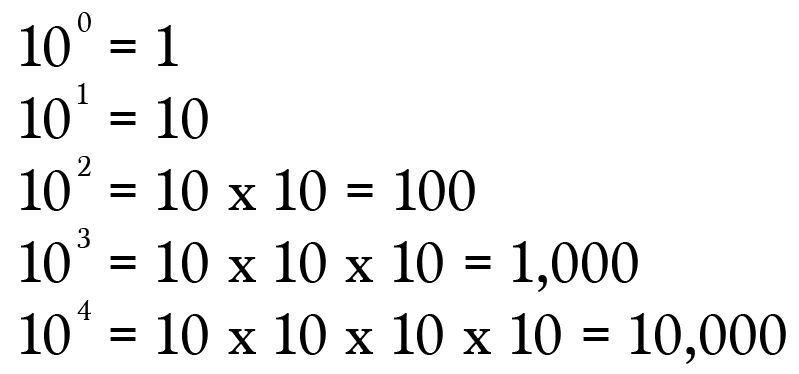
Keep the exponent pattern to the correct of the decimal point, subtracting 1 from the exponent of each place-value.

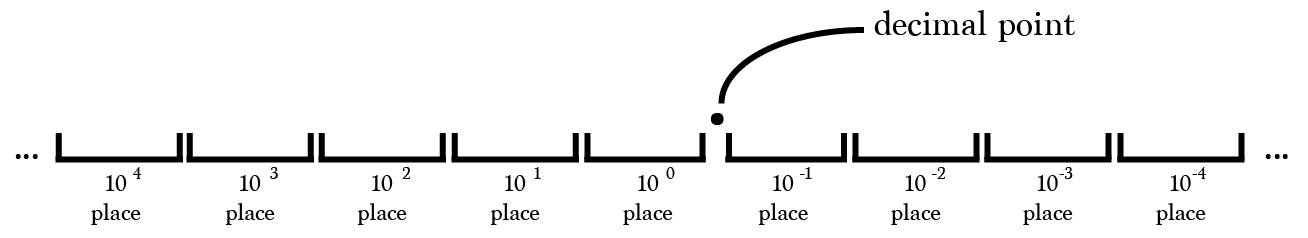
diagram two: decimal system with exponent labels

We now have negative exponents! How awesome is that?!

Compare the exponent representation in diagram ii to the traditional representation in diagram 1. What patterns do you detect?

You might notice that the 2nd and 3rd column are very similar. This is an important insight regarding negative exponents.

Negative exponents limited how many times 1 must exist divided by the base of operations number.

Example

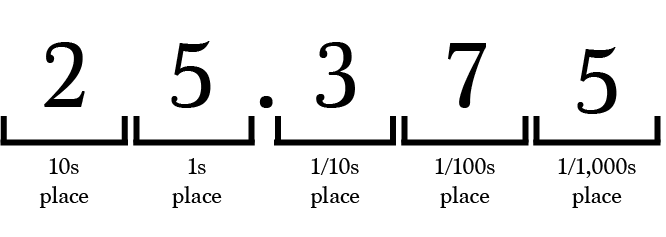
Using the place-value assignments, 25.375 is equivalent to 2 tens, 5 ones, 3 tenths, 7 hundredths and five thousandths. We can express this in expanded class using exponent notation.

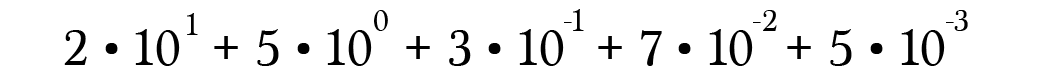
Or only past the equivalent of each place-value.

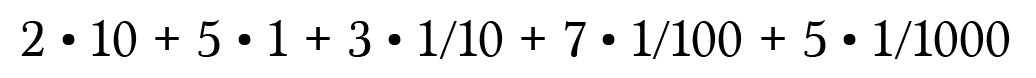
If we multiply the products together and perform the addition, we arrive back at the original value.

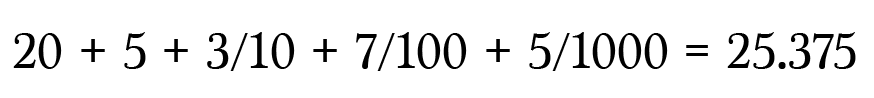
Reciprocals

At this indicate you may exist wondering:

Is in that location a relationship between positive and negative exponents?

At that place is! They are called reciprocals or multiplicative inverses considering when multiplied together they result in ane. To demonstrate, we'll multiply the following two values together.

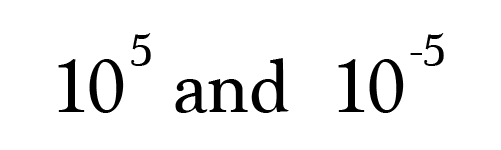
A negative exponent can be rewritten with an inversion:

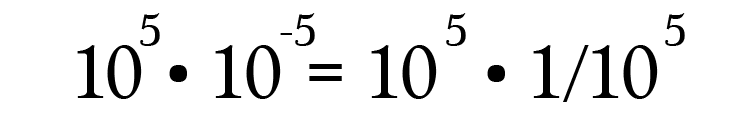
Next, aggrandize both exponents and simplify the answer. That proves they are reciprocals of one another.

For more on Negative Exponents, including how to simplify algebraic expressions involving negative exponents, check out the video above or cheque out the Math Hacks YouTube aqueduct!